Hej! Today we will talk about something in geometry called a linear pair of angles. It might sound a little scary, but don't worry! We will break it down step by step.
What is an Angle?
First, we need to know what an angle is. Imagine two lines or line segments that meet at a point. That point is called the vertex. The space between those two lines is the angle.
Think of a clock. The hands form an angle! When the clock shows 3:00, the angle between the hour and minute hand is a right angle (90 degrees). Angles are usually measured in degrees.
Angles have names! We often name them using three points: one on each line forming the angle and the vertex in the middle. For example, if we have an angle with vertex 'L' and points 'K' and 'M' on the lines, we can call it angle KLM or angle MLK. The middle letter ALWAYS represents the vertex, or corner, of the angle. We use the symbol ∠ to denote an angle, so ∠KLM represents angle KLM.
What is a Line?
Next, let's define what a line is. A line is a straight path that goes on forever in both directions. Think of a perfectly straight road that doesn't end.
A line can be represented as a line segment with arrows on both ends to indicate it continues infinitely. We usually name a line with two points on the line, for example, line KM. In geometry, a straight line forms an angle of 180 degrees. This is also called a straight angle.
Consider the edge of your desk. If it goes on forever in both directions, that would be a line! We need to remember that a line represents 180 degrees because it's crucial for understanding linear pairs.
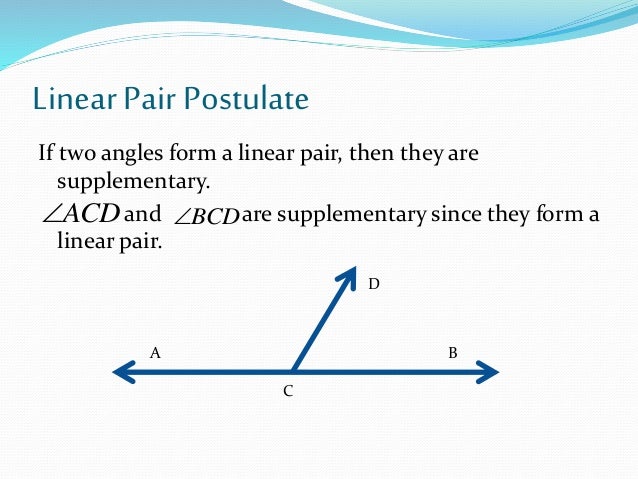
Introducing the Linear Pair
Now we're ready to talk about a linear pair of angles. Two angles are called a linear pair if they share a common side and their non-common sides form a straight line.
Imagine two pieces of pie cut from a circular pie, but only half the pie is left. The two slices share the cut in the middle, and together they form a half-circle. The angles of those pie slices form a linear pair! Together, they make the straight edge of the remaining half-pie. That is the core idea to understand what forms a linear pair of angles.
Another easy way to think of it is to imagine a line. Then, draw another line that starts from a point on the first line and goes in a different direction. The two angles created at that point are a linear pair. It's like splitting a straight line into two angles.
Key Property of Linear Pairs
The most important thing about a linear pair is that the sum of the measures of the two angles is always 180 degrees. This is because they form a straight line!
If you have one angle of a linear pair, you can always find the other angle by subtracting the known angle from 180 degrees. For example, if one angle is 60 degrees, the other angle in the linear pair must be 180 - 60 = 120 degrees.
This property is super useful when solving geometry problems! If you know the angles are a linear pair, you immediately know their sum is 180 degrees. Knowing this relationship unlocks many problem solving opportunities.
Angle KLM and Angle MLN: An Example
Let's use the example of angle KLM and angle MLN. Let's assume that point 'L' is on line KN. Let's also assume that point M is somewhere off of that line. In this case, angles KLM and MLN are a linear pair.
Why? Because they share a common side: LM. Also, their non-common sides, KL and LN, form the straight line KN.
Therefore, we know that ∠KLM + ∠MLN = 180 degrees. If ∠KLM is 70 degrees, then ∠MLN must be 110 degrees (180 - 70 = 110). If ∠MLN is 35 degrees, then ∠KLM must be 145 degrees (180 - 35 = 145). See how this can work?
Let's Look at Some Scenarios
Imagine a seesaw. When one side goes up, the other goes down. Let's call the pivot point 'L'. Let's call one end 'K' and the other 'N'. If you drew a line from K to L and then from L to N, you would have a straight line. Now imagine a point 'M' somewhere between the seesaw and the ground. Draw lines from K to M and N to M. This creates angles ∠KLM and ∠MLN. These are NOT necessarily a linear pair because KLN does NOT form a straight line.
However, if we think of the flat ground as the line KN, and then imagine a stick leaning against something at point 'L', forming the line LM. Then the angles formed at 'L' ARE a linear pair. As the stick leans closer to the ground, one angle gets smaller, and the other gets bigger, but their sum always remains 180 degrees!
Think about a door opening. The edge of the door and the wall where the door is connected form a straight line. When the door opens, it creates two angles: one between the door and the wall on one side, and one between the door and the wall on the other side. These angles form a linear pair.
Why is this Important?
Understanding linear pairs is crucial for learning more advanced geometry concepts. It's a foundational idea that will help you solve problems involving angles, lines, and shapes.
You'll often use the fact that linear pairs add up to 180 degrees to find missing angles in triangles, quadrilaterals, and other polygons. It's a tool in your geometry toolbox!
Furthermore, understanding linear pairs can help in everyday situations. From construction to design, recognizing these relationships can be surprisingly useful. For example, when building a roof, understanding the angles needed to create the correct slope is crucial, and often relies on the principles of linear pairs and supplementary angles.
In Summary
Let's recap! An angle is formed by two lines or line segments meeting at a vertex. A line is a straight path that goes on forever. A linear pair of angles consists of two angles that share a common side and whose non-common sides form a straight line.
The key property of a linear pair is that the sum of the measures of the two angles is always 180 degrees. ∠KLM and ∠MLN are a linear pair if they share the side LM, and KL and LN form a straight line. Therefore, ∠KLM + ∠MLN = 180 degrees.
With practice and a little bit of visualization, you'll master the concept of linear pairs in no time! You've got this!