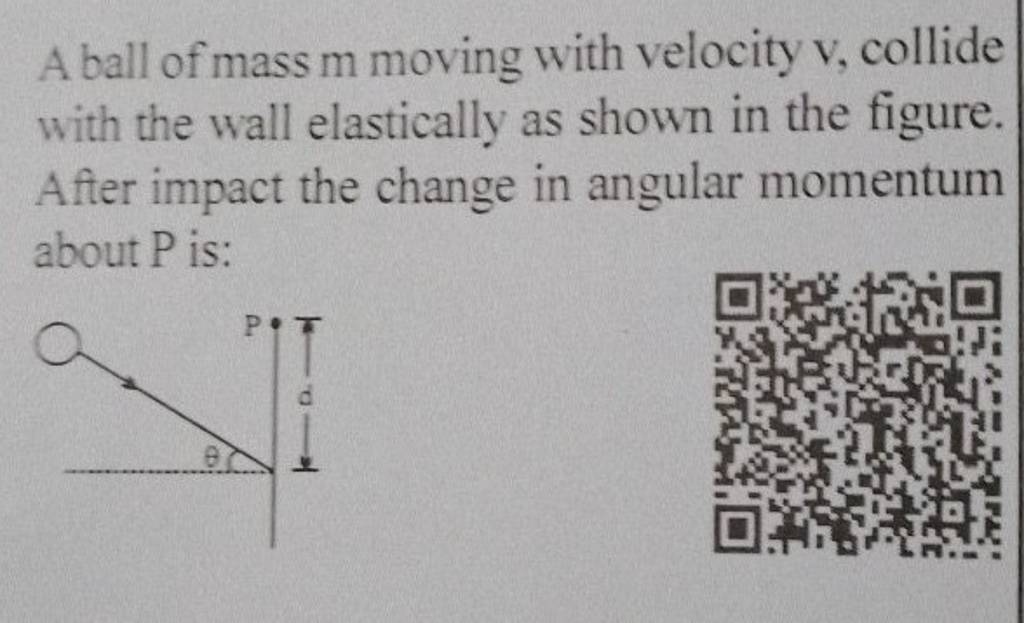
Hej! Przygotujmy się razem do egzaminu z fizyki. Skupimy się na czymś konkretnym: kulce o masie M, poruszającej się z prędkością V.
Energia kinetyczna
Zacznijmy od energii kinetycznej. Jest to energia, którą obiekt posiada dzięki swojemu ruchowi.
Energia kinetyczna oznaczana jest symbolem Ek. Dla naszej kulki, Ek obliczamy ze wzoru: Ek = (1/2) * M * V2.
M to masa kulki (w kilogramach). V to prędkość kulki (w metrach na sekundę). Ważne są jednostki!
Pamiętaj, że prędkość podnosimy do kwadratu. Większa prędkość oznacza znacznie większą energię kinetyczną!
Przykład
Załóżmy, że M = 2 kg, a V = 3 m/s. Wtedy Ek = (1/2) * 2 kg * (3 m/s)2 = 9 J. Jednostką energii jest dżul (J).
Spróbuj teraz sam. Co się stanie z Ek, jeśli zwiększymy V do 6 m/s?
Pęd
Kolejnym ważnym pojęciem jest pęd. To miara "ilości ruchu" danego obiektu.
Pęd oznaczamy symbolem p. Dla naszej kulki, p = M * V.
M to nadal masa, a V to prędkość. Tym razem nie podnosimy prędkości do kwadratu!
Pęd jest wektorem. Ma zarówno wartość, jak i kierunek. Kierunek pędu jest taki sam jak kierunek prędkości.
Jednostki
Jednostką pędu jest kilogram razy metr na sekundę (kg * m/s).
Przykład
Dla M = 2 kg i V = 3 m/s, pęd p = 2 kg * 3 m/s = 6 kg * m/s.
Co się stanie z pędem, jeśli masa kulki wzrośnie?
Zasada zachowania pędu
Jedną z najważniejszych zasad w fizyce jest zasada zachowania pędu. Mówi ona, że w układzie izolowanym całkowity pęd układu pozostaje stały.
Układ izolowany to taki, na który nie działają siły zewnętrzne.
Oznacza to, że jeśli kulka zderzy się z innym obiektem, całkowity pęd kulki i tego obiektu przed zderzeniem będzie równy całkowitemu pędowi po zderzeniu.
Zastosowanie
Zasada zachowania pędu jest bardzo przydatna do analizy zderzeń i wybuchów.
Wyobraź sobie, że kulka zderza się z nieruchomą kulą o masie m. Po zderzeniu obie kulki poruszają się razem z prędkością v. Możemy obliczyć v, korzystając z zasady zachowania pędu.
Przed zderzeniem pęd kulki M wynosił M * V, a pęd kuli m wynosił 0. Po zderzeniu pęd obu kulek wynosi (M + m) * v. Zatem M * V = (M + m) * v. Możemy wyznaczyć v: v = (M * V) / (M + m).
Praca
Praca to energia potrzebna do przesunięcia obiektu na pewną odległość, działając siłą. Oznaczamy ją symbolem W.
Jeśli siła F działa na kulkę na odległości d w kierunku ruchu, to praca W = F * d.
Jednostką pracy jest dżul (J).
Związek z energią kinetyczną
Praca wykonana nad kulką może zmienić jej energię kinetyczną. Mówi o tym twierdzenie o pracy i energii.
Jeśli praca W jest wykonana nad kulką, to zmiana jej energii kinetycznej jest równa tej pracy: W = ΔEk = Ek końcowa - Ek początkowa.
Przykład
Siła F działająca na kulkę o masie M, powoduje, że jej prędkość wzrasta z V1 do V2 na drodze d. Wtedy F * d = (1/2) * M * V22 - (1/2) * M * V12.
Podsumowanie
Zapamiętaj kluczowe pojęcia:
- Energia kinetyczna (Ek): Ek = (1/2) * M * V2
- Pęd (p): p = M * V
- Zasada zachowania pędu: W układzie izolowanym całkowity pęd pozostaje stały.
- Praca (W): W = F * d
- Twierdzenie o pracy i energii: W = ΔEk
Ćwicz rozwiązywanie zadań. Im więcej zadań zrobisz, tym lepiej zrozumiesz te pojęcia.
Powodzenia na egzaminie! Wierzę w Ciebie!